Fttx-The Last Miles,Fttx - Last Mile Networking Solution,Last-Mile Otdr For Fttx Networks Integrates Opm,Last Mile Fttx Test Huizhou Fibercan Industrial Co.Ltd , https://www.fibercaniot.com
This application note describes how to add margin adjustment in a DC-DC converter by connecting a 4-channel adjustable current DAC DS4404 (or DS4402's 2-channel version DS4402).
It can be seen from the circuit shown in Figure 1 that adding a DS4404 to the existing design is very simple. DS4404 is added to the feedback node of the DC-DC converter (see dashed line) to adjust the VOUT of the DC-DC converter. At power-up, the output current of the DS4404 is 0A (presented as a high-impedance state). Before writing data through the I²C interface, the DS4404 is in a transparent state. 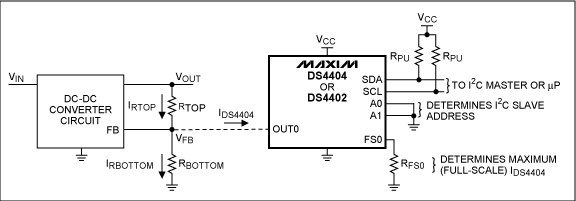
Figure 1. Connection of DS4404 in the feedback circuit of a DC-DC converter
The following assumptions are made in the example (not related to DS4404):
VIN = 3V to 5.5V
VOUT = 1.8V (required nominal output voltage)
VFB = 0.6V (not to be confused with DS4404's VREF)
The VFB value can be obtained in the data sheet of the DC-DC converter, confirm that the voltage is within the OUTx voltage range (VOUT: SINK and VOUT: SOURCE) specified in the DS4404 data sheet. Finally, it is also important to verify the input impedance of the FB pin of the DC-DC converter. This example assumes that the pin input is high impedance.
Assuming that DS4404 is used to add ± 20% margin adjustment to VOUT, we can get:
VOUTMAX = 2.16V
VOUTNOM = 1.8V
VOUTMIN = 1.44V
First determine the relationship between RTOP and RBOTTOM. When IDS4404 = 0A, get the nominal value of output voltage VOUT VOUTNOM. 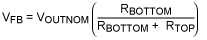
Solve the above formula to get RTOP: 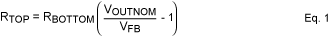
For this example: 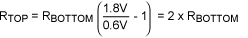
The current IDS4404 required to increase VOUT to VOUTMAX can be obtained by the sum of the FB node currents. 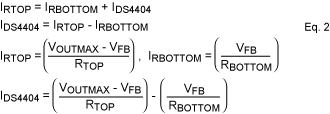
RBOTTOM can be obtained by solving Equation 1, and the above equation can be simplified to: 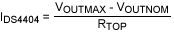
Or expressed by the percentage of adjustment margin: 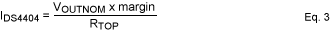
For the margin of ± 20% in this example, margin = 0.2 in the above formula.
Of course, before using the above relationship to calculate RTOP and RBOTTOM, you must first select the full-scale current IFS.
According to the DS4404 data sheet, full-scale current between 0.5mA and 2.0mA (defined as IOUT: SINK and IOUT: SOURCE in the DS4404 data sheet, depending on the sink current or source current) can ensure accuracy and linearity. Unfortunately, there is no equation available to calculate the ideal full-scale current, and different applications will yield different results. The factors that affect the selection of full-scale current are: adjustment level, step size, RTOP, RBOTTOM. Similarly, when using a specific register to set the corresponding margin percentage requirements, there may be other factors. In any case, selecting the ideal full-scale current requires repeated testing to determine RTOP, RBOTTOM, RFS, and step size. Once the full-scale current is determined, it can be adjusted or some register values ​​can be adjusted to allow the use of common resistance values.
Returning to the original example, calculating RTOP such that IFS = IDS4404, provides 31 steps from VOUTNOM to VOUTMAX, and also provides 31 steps from VOUTNOM to VOUTMIN, enough to meet the requirements of this example.
In addition, we can also arbitrarily choose an IFS as the center point of the specified range (1.25mA), and then perform the calculation. As a demonstration, we will calculate the endpoints of the range.
For: IFS = IDS4404 = 0.5mA.
Use Equation 3 to derive the RTOP: 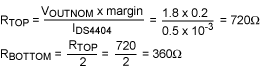
Using the formula and VREF value in the DS4404 data sheet, the RFS can be calculated. For details, please refer to the DS4404 data sheet. 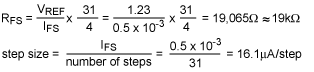
Finally, the output current of the DS4404 is completely determined as a function of the register setting: 
Note: The register setting here does not include the sign bit. The sign bit is usually used to select the current sink or source current. When the sign bit = 0, the DS4404 output is sinking current, increasing VOUT to VOUTMAX; when the sign bit = 1, the DS4404 output is the source current, reducing VOUT to VOUTMIN.
Similarly, when IFS = IDS4404 = 2.0mA: 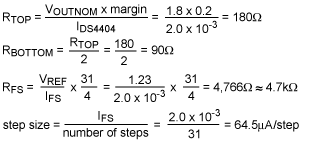
Comparing RTOP and RBOTTOM, it can be seen that the impedance is higher when IFS = 0.5mA, which is more advantageous.
How to add margin adjustment function in DC-DC converter
Abstract: This application note describes a simple method to add margin adjustment function in a DC-DC converter by connecting a DS4404 4-channel adjustable current DAC (or 2-channel version DS4402).